Max Planck Institute for Medical Research

Back projection and r-weighted back projection

Axial Tomography by filtered least squares

The Eigenfunctions

Fig 3: Each of the eigenvectors of HTH (one of the the columns of V) may be mapped onto the lattice since each component of the vector is associated with a lattice point. When we do this we generate two-dimensional functions in the circular space we have chosen for the solution. These functions are (by definition) orthonormal. Because we have chosen a circular boundary, they look rather like cylinder functions. In fact they have a symmetry characteristic of the geometry of the problem. — Fig 3: Each of the eigenvectors of H^TH (one of the the columns of V) may be mapped onto the lattice since each component of the vector is associated with a lattice point. When we do this we generate two-dimensional functions in the circular space we have chosen for the solution. These functions are (by definition) orthonormal. Because we have chosen a circular boundary, they look rather like cylinder functions. In fact they have a symmetry characteristic of the geometry of the problem.

© Kenneth C. Holmes

Fig 3: Each of the eigenvectors of H^TH (one of the the columns of V) may be mapped onto the lattice since each component of the vector is associated with a lattice point. When we do this we generate two-dimensional functions in the circular space we have chosen for the solution. These functions are (by definition) orthonormal. Because we have chosen a circular boundary, they look rather like cylinder functions. In fact they have a symmetry characteristic of the geometry of the problem.

© Kenneth C. Holmes

Fig 3: Each of the eigenvectors of HTH (one of the the columns of V) may be mapped onto the lattice since each component of the vector is associated with a lattice point. When we do this we generate two-dimensional functions in the circular space we have chosen for the solution. These functions are (by definition) orthonormal. Because we have chosen a circular boundary, they look rather like cylinder functions. In fact they have a symmetry characteristic of the geometry of the problem. — Fig 3: Each of the eigenvectors of H^TH (one of the the columns of V) may be mapped onto the lattice since each component of the vector is associated with a lattice point. When we do this we generate two-dimensional functions in the circular space we have chosen for the solution. These functions are (by definition) orthonormal. Because we have chosen a circular boundary, they look rather like cylinder functions. In fact they have a symmetry characteristic of the geometry of the problem.

© Kenneth C. Holmes

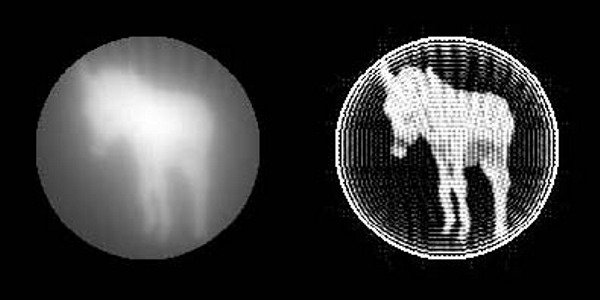
Fig 3: Each of the eigenvectors of H^TH (one of the the columns of V) may be mapped onto the lattice since each component of the vector is associated with a lattice point. When we do this we generate two-dimensional functions in the circular space we have chosen for the solution. These functions are (by definition) orthonormal. Because we have chosen a circular boundary, they look rather like cylinder functions. In fact they have a symmetry characteristic of the geometry of the problem.

© Kenneth C. Holmes

Each of the eigenvectors of H^TH (one of the the columns of V) may be mapped onto the lattice since each component of the vector is associated with a lattice point. When we do this we generate two-dimensional functions in the circular space we have chosen for the solution. These functions are (by definition) orthonormal. Because we have chosen a circular boundary, they look rather like cylinder functions. In fact they have a symmetry characteristic of the geometry of the problem. Some examples from a case considered below are shown in Fig 3.

Contact

Kenneth C. Holmes

+49 6221 486-437 ken.holmes@...

http://www.mpimf-heidelberg.mpg.de/~holmes/

References

Klug, A.; Crowther, R. A.

Three-dimensional image reconstruction from the viewpoint of information theory

Nature 238, 435 - 440 (1972)

Gilbert, P.

Ph.D. Thesis

University of Cambridge (1972)

Crowther, R. A.; DeRosier, D. J.; Klug, A.

The reconstruction of a three-dimensional structure from projections and its application to electron microscopy

Proc. R. Soc. Lond. A 317 (1530) 319 - 340 (1970)

Diamond, R.

Filtering in the Method of Least-Squares

Acta Cryst. B 25, 805 (1969)

Holmes, K. C.; Angert, I.; Kull, F. J.; Jahn, W.; Schröder, R. R.

Electron cryo-microscopy shows how strong binding of myosin to actin releases nucleotide

Nature 425, 423 - 427 (2003)